Half the area of an L
Two arbitrary rectangles are placed to form an “L”. That is, the lower left-hand corner of the two rectangles share the same point. (What I’m trying to say is that there’s an “L” whose “I” and “_” parts have arbitrary widths and heights.) Using only a (pen and a) straightedge (that is, no measuring device and no compass), figure out a way to, with a single straight cut, divide the “L” into two pieces of equal area.
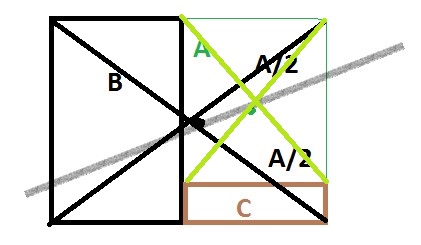
draw a vertical line from the right bottom of the L-shape and a horizontal line from the left top of the L-shape do that this two lines meet each other.
This gives us 3 squares: the right lower part of the L-shape (lets call it rectangle C), The vertical rectangle of the L-shape (lets call it rectangle B), and the new rectangle between them (let call it rectangle A).
Since Any line passing through the center of a rectangle divides it in half we find the center of rectangle A by drawing the intersecting diagonals.
We do the same for the square made up from the 3 rectangles (A,,B,C), and so the line passing threw 2 two points divides the L-shape into 2 equal parts.
Your Answer
More puzzles to try-

What is the logic behind these ?
3 + 3 = 3 5 + 4 = 4 1 + 0 = 3 2 + 3 = 4 ...Read More »
Defective stack of coins puzzle
There are 10 stacks of 10 coins each. Each coin weights 10 gms. However, one stack of coins is defective ...Read More »
Which clock works best?
Which clock works best? The one that loses a minute a day or the one that doesn’t work at all?Read More »
(Advanced) Cheryl’s Birthday Puzzle
Paul, Sam and Dean are assigned the task of figuring out two numbers. They get the following information: Both numbers ...Read More »
Five greedy pirates and gold coin distribution Puzzle
Five puzzleFry ship’s pirates have obtained 100 gold coins and have to divide up the loot. The pirates are all ...Read More »
Magical flowers!!
A devotee goes to three temples, temple1, temple2 and temple3 one after the other. In front of each temple, there ...Read More »
Tuesday, Thursday what are other two days staring with T?
Four days are there which start with the letter ‘T‘. I can remember only two of them as “Tuesday , Thursday”. ...Read More »
How could only 3 apples left
Two fathers took their sons to a fruit stall. Each man and son bought an apple, But when they returned ...Read More »
How Many Eggs ?
A farmer is taking her eggs to the market in a cart, but she hits a pothole, which knocks over ...Read More »
HARD MATHS – How much faster is one train from other Puzzle
Two trains starting at same time, one from Bangalore to Mysore and other in opposite direction arrive at their destination ...Read More »
Most Analytical GOOGLE INTERVIEW Question Revealed
Let it be simple and as direct as possible. Interviewer : Tell me how much time (in days) and money would ...Read More »
Lateral thinking sequence Puzzle
Solve this logic sequence puzzle by the correct digit- 8080 = 6 1357 = 0 2022 = 1 1999 = ...Read More »
How did he know?
A man leaves his house in the morning to go to office and kisses his wife. In the evening on ...Read More »
Pizza Cost Math Brain Teaser
Jasmine, Thibault, and Noah were having a night out and decided to order a pizza for $10. It turned out ...Read More »
Which letter replaces the question mark
Which letter replaces the question markRead More »
Which room is safest puzzle
A murderer is condemned to death. He has to choose between three rooms. The first is full of raging fires, ...Read More »
Richie’s Number System
Richie established a very strange number system. According to her claim for different combination of 0 and 2 you will ...Read More »
Srabon wanted to pass
The result of math class test came out. Fariha’s mark was an even number. Srabon got a prime!! Nabila got ...Read More »
Become Normal!!
Robi is a very serious student. On the first day of this year his seriousness for study was 1 hour. ...Read More »
Sakib Knows The Number!
Ragib: I got digits of a 2 digit number Sakib: Is it an odd? Ragib: Yes. Moreover, the sum of ...Read More »



